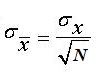Recall from the Central Limit Theorem that the standard error of the mean is equal to the population standard deviation, s , divided by the square root of the sample size:
Thus, for a sample of N = 25 and population standard deviation of s = 100, the standard error of the mean is 100/5 or 20.
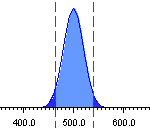
We know that approximately 95% of scores in a normal distribution are within two standard deviations of the mean (1.96 standard deviations, to be more precise). If the sampling distribution of means is normal, then we expect about 95% of means from samples with N = 25 to be within about 39 points of the actual population mean (1.96 * 20 = 39.2), from about 461 to 539 (see figure below).
![]()