The applet below allows us to simulate drawing random samples of VAST test takers from a normally distributed null population with a mean (m) of 500 and a standard deviation (s) of 100.
1. To begin, use the drop down menu under Sample size: and select N=25. Under Show:, check Show sample data and Show population (with no other boxes checked for now).
2. Click Draw a sample to draw a random sample of 25 cases from this population. The individual scores are represented by black boxes and the sample mean is shown with a red arrow.
3. Click Draw a sample at least ten times to observe how a sample mean based on N = 25 is likely to be quite close to the population mean of 500.
4. Click Show obtained means to see your observed sample means.
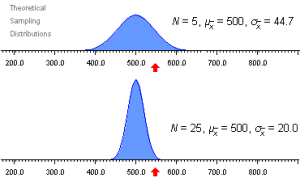
5. To view the theoretical distribution of all possible sample means based on samples of N = 25 selected randomly from the Null Population, click Show sampling distribution of the mean. The standard deviation of this distribution is the standard error of the mean, shown in the box to be equal to 20.00, the value we computed earlier. Click here to review the computation.
6. To observe the sampling distribution of means for smaller samples with N = 5, use the drop down menu under Sample size: and select N=5. Notice that the distribution of possible means has greater variability. The standard error of the mean is reported in the box to be 44.72. Review computation.
Question C: Sampling Error
From least to most likely, rank the following events (assume random sampling from the population of VAST-test takers with m = 500 and s = 100):
- Obtaining an individual score of 550 or greater.
- Obtaining a sample mean of 550 or greater with a sample size of 25.
- Obtaining a sample mean of 550 or greater with a sample size of 5.
1, 2, 3
Incorrect! Compare the sampling distributions of means for samples of size N with the sampling distribution of means for samples with N = 25. Where does a value of 550 fall within each distribution? Notice that the farther a value falls in the tails, the less likely it is. The distribution for individual scores is the original null distribution with mean = 500 and SD = 100. This is equivalent to the sampling distribution for samples of size N = 1. Compared to the original null population distribution, is 550 farther in the tail or closer to the center for the the two distributions shown below?
3, 2, 1
Incorrect! Compare the distributions of sample means for N = 5 and for N = 25. For which sample size would a sample mean of 550 be less likely (further in the tail of the distribution)?

3, 2, 1
Correct! With smaller samples, a sample mean is likely to deviate more from the population mean. Not surprisingly, larger samples produce means that are likely to cluster more closely around the population mean. The smallest sample is a single observation, N = 1. When N = 1, the sampling distribution of the mean is equivalent to the population distribution.
Events equally likely.
Incorrect! Compare where a value of 550 falls within each of the distributions. The farther a value is in the extremes of the tails of the distribution, the less likely it is.

![]()

