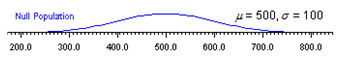
The Verbal Ability and Skills Test (VAST) is a standardized test with a range of 0 to 1000. VAST scores are normally distributed within the population of test takers with a mean score, m, of 500 with a standard deviation, s, of 100. The blue curve shows the distribution of this population of scores.
The ACE training program boasts that their graduates score higher on average than the population of individuals who do not participate in a training course (the null population). That is, ACE claims test takers who first complete the ACE training program on average score higher than 500 on the VAST.
We will test their claim by comparing the mean of a random sample of ACE graduates to the null population mean of 500. Hypothesis testing procedures allow us to compute the probability of finding a sample mean as large as we observed if the training had no effect.
Question B: Convincing Evidence
What would convince you that graduates of ACE do indeed have average test scores greater than 500?
Question B1: Would you be convinced that the average score for ACE graduates is greater than 500 if you were told that one randomly selected ACE graduate had a score of 550? (What is the probability that a randomly sampled score from the normally distributed population is 550 or greater, given that the population mean is 500 and the standard deviation is 100?)
Answer (think about your own answer before clicking here to view our answer)
Our Answer for QB1: No. Even if ACE training has no effect it would not be surprising to find that one randomly selected ACE graduate has a score 50 points greater than the null population mean of 500. The population standard deviation is 100, so a score of 550 is only .50 standard deviations greater than the null population mean (z = .50). Given that the distribution is normal, we can use a normal distribution table or the WISE p–zConverter to find the probability that a randomly selected score is .50 or more standard deviations greater than the mean. This probability is .309, indicating that about 31% of the time, a randomly selected individual would have a VAST score of 550 or greater (see the null population distribution above and verify this).
Question B2: Would it be more convincing if 25 randomly selected ACE graduates had an average score of 550? Why?
Answer
Our Answer for QB2: Yes. From the Central Limit Theorem we know that the distribution of sample means (i.e., the ‘sampling distribution of the mean’) has less variability than individual scores, so a sample mean 50 points greater than the population mean of 500 is stronger evidence in support of ACE than a single score 50 points greater than the mean.
Question B3: What is the numerical value for the standard error of the mean for a sample of N = 25 drawn randomly from a population with a standard deviation of s = 100? (See general formula for calculating the standard error.)
Answer
Our Answer for QB3:
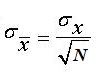
For a sample of N = 25 drawn randomly from a population with a standard deviation of s = 100, the standard error of the mean is 100/5 or 20.
If you are unsure about answers to these questions, you may find it helpful to complete the WISE tutorials on the sampling distribution of the mean and Central Limit Theorem before proceeding with this tutorial on hypothesis testing.
- The principles and procedures of hypothesis testing allow us to compute how unlikely our observed data are if the mean really is 500 in the population from which our sample is drawn.
![]()

