Calculating z-scores
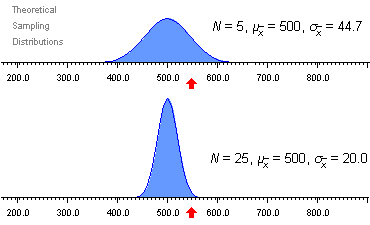
In the previous question, we saw that obtaining a sample mean of 550 or greater from a random sample of VAST-test takers is more likely when the sample size is 5 than when the sample size is 25. How likely is each outcome? To find the exact probabilities, we can calculate the z-score associated with a sample mean of 550 with respect to the sampling distributions of means for each sample size and then use the z distribution to find the probability, or p-value.
The formula to convert a sample mean, X, to a z-score is:
where μ is the population mean, σ is the population standard deviation, and N is the sample size.
From z-scores to p-values
Looking at the numerator (top portion) in the formula, we can see that the more the sample mean deviates from the population mean, the bigger the z-score. Also notice when the denominator (bottom portion, which is the standard error of the mean) is smaller, the obtained z-score is bigger. Bigger or more extreme z-scores are associated with smaller p-values, meaning they are less probable.
With a sample size of N = 25 VAST-test takers, the z-score that corresponds to a sample mean of X = 550 is:
Using the p–z converter, this z-score of 2.5 corresponds to a p-value of .006. That is, when drawing a random sample of 25 VAST-test takers, the probability of obtaining a sample mean of 550 or greater is 0.6%—a very unlikely event.
What happens with a smaller sample size? How much more likely is it to obtain a sample mean of 550 if we have a sample with N = 5? Answer the next question to find out.
Question D: z-scores and Probabilities
VAST scores are normally distributed with a mean score of 500 and a standard deviation of 100. Calculate the z-score and the probability of obtaining a sample mean 550 or greater when N = 5 (assume random sampling; you can compute the z-score and use the p–z converter to convert the z-score into a p-value).
z-score is: p-value is:
Show me a hint
Recall that z-scores reflect how much a score deviates from the mean. To convert a sample mean Xinto a z-score, subtract the population mean, m, from X and then divide by the standard error of the mean (s divided by the square root of N):
The mean of VAST scores is 500 and the standard deviation is 100. With a sample size of 5, a sample mean of 550 equals a z-score of 1.12. What is the associated probability of this z-score? (You use the p–z converter to find the probability of obtaining a z-score this large or larger.)
Check Answer
With a sample size of 5, the probability of obtaining a sample mean that is 550 or greater is about 13.1% (z = 1.12, p = .131), which is much more likely than when the sample size is 25 (p = .006).
z-score |
One-tailed
|
|
| A sample mean of 550 or greater when N = 5 | 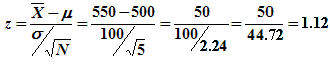 |
.131 |
| A sample mean of 550 or greater when N = 25 | 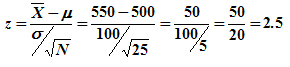 |
.006 |
Notice how the probabilities of 13.1% and 0.6% are related to where 550 falls within the different sampling distributions:
![]()