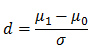
1f. I think that with a smaller standard deviation in the population, the statistical power will be:
Less
Try again. A smaller standard deviation means less variability.
Unchanged
Try again.
Greater
Correct! Assuming no other population values change, as the variability of the population decreases, power increases. Watch what happens in the applet when variability is changed. Later you will be asked to explain why this is the case.
I don’t know.
Hint: Look at the formula above. As standard deviation increases, what happens to the effect size?
To simulate drawing a sample from graduates of the TREY program that has the same population mean as the DEUCE program (520), but a smaller standard deviation (50 instead of 100), enter the following values into the WISE Power Applet:
Press enter/return after placing the new values in the appropriate boxes.
Do three simulations of drawing a sample of 25 cases and record the results below.
| Trial | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
|---|---|---|---|---|---|---|---|---|---|---|
| Mean | 512.1 | 516.4 | 515.6 | 515.4 | 525.2 | 535.3 | 528.6 | |||
| Z-Score | 1.21 | 1.64 | 1.56 | 1.36 | 2.52 | 3.53 | 2.86 |
1g. How many of your ten simulated samples allowed you to reject the null hypothesis?
(Use one-tailed alpha α = .05, z = 1.645, so reject H0 if your z-score is greater than 1.645)
1h. What is the power for this test (from the applet)?
(Click here to see how power can be computed for this scenario.)
1i. In Exercise 1b the DEUCE program had a mean of 520 just like the TREY program, but with samples of N = 25 for both programs, the test for the DEUCE program had a power of .260 rather than .639. The standard deviation for DEUCE was 100 rather than 50. Why is statistical power greater for the TREY program?
Because smaller population variance always produces greater power.
Try again.
Because the program with the larger effect size always produces greater power.
Try again.
Neither of these reasons is sufficient.
Correct! Remember BEAN – when assessing power, we need to consider E, A, and N. Smaller population variance or larger effect size doesn’t guarantee greater power if, for example, the sample size is much smaller. If sample size and alpha are not changed, then the power is greater if the effect size is larger. In the current example, the effect size for the DEUCE program was 20/100 = 0.20 while the effect size for the TREY program was 20/50 = 0.40. If nothing else differs, the program with the larger effect size has the greater power because more of the sampling distribution for the alternate population exceeds the critical value.
![]()

